Compiled by AnjulberG for non-profit classroom use. Includes material from various sources |
INSTRUCTIONS: You must do at least two (2) problems from each module.Each question is worth either 1, 2 or 3 credits -  . .You must score at least 10 credits to complete your contract. You can do more problems to get more credits, of course. |
MODULE ONE
(1) Balanced or What?! How many 'C' balls does it take to balance one 'A' ball? [Help] 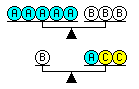
|
(2) Pencil and Pen Problems. Jenny wanted to purchase 2 dozen pencils and a pen. Those items cost $8.45 and she did not have enough money. So she decided to purchase 8 fewer pencils and paid $6.05. How much was a pen? [Help] |
|
(3) Money Trouble Bob, Jim and Cathy each have some money. The sum of Bob's and Jim's money is $18.00. The sum of Jim's and Cathy's money is $21.00. The sum of Bob's and Cathy's money is $23.00. How much money does each person have? [Help] |
(4) Age-old Problem Tom's mother is 30 years old. The three children are 5, 3, and 0 years old. 12 years later, the total age of Tom's mother and father is twice as much as the total of the ages of all three children. How old is Tom's father now? [Help] |
MODULE TWO
|
(5) ROWLING J. K. Rowling, author of the Harry Potter books, began a British tour Saturday at King's Cross train station in Central London on Platform 9-3/4. The tour is to celebrate her latest release, Harry Potter and the Goblet of Fire. In reality, there is no platform 9-3/4, except in the fictional world of Harry Potter. But let's leave reality and assume that platforms are labelled in quarter-number intervals. If the first is platform 1 and the last is platform 15, how many platforms are there total? [Help] |
(6) Cyclist After a cyclist has gone two thirds of her journey, she gets a puncture and must walk the last third of her journey. Finishing on foot, she spends twice as long walking as she did riding. How many times as fast does she walk as ride? [Help]  |
|
(7) More Trains! A train moving at 60km/hour meets and is passed by a train moving at 75km/hour. A passenger in the first train sees the second train take 8 seconds to pass him by. How long is the second train? [Help]  |
(8) BART the PAPER BOY Bart Simpson decided to make a graph of his weekly earning of $120.00 from his paper route. To make the size of each sector proportional to the amount distributed what does the angle x in degrees have to be? [Help] 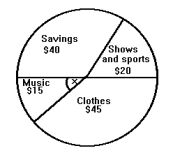 |
MODULE THREE
|
(9) Calendar Cubes 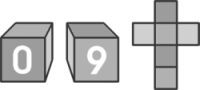
Make a pair of cubes that can be moved to show all the days of the month from the 1st to the 31st. For example, the cubes above show the 9th. (10) Queen Quiz Show how you could place 8 queens on a chessboard so that no queen could capture another queen on the next move. All positions are ON the field of play! [Help] |
(11) Egyptian Rope Problems 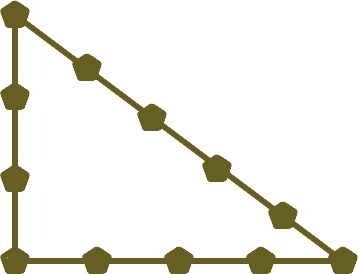 The ancient Egyptians were said to make right-angled triangles using a rope with 12 equal sections divided by knots. Show how this rope can be used to make an isosceles and equilateral triangle, and three other regular shapes.They must have a knot at each corner. Regular shapes are shapes with congruent sides Each corner must be on a knot!!! [Help] |
|
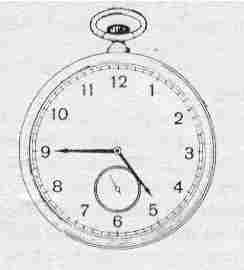
(12) Meet Your Match The drawing below shows four squares made by using 12 matches. Move 4 matches and still use all 12 matches to make 3 squares. You may not break the matches. Each side of each square must be made up of one match - you canít have matches lying side-by-side! 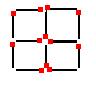 (13) Watch It!!! Divide the watch face with 2 straight lines so that the sums of the numbers in each part are equal. |
(14) Soma Easy, Soma Hard!!! Use soma cubes to make 2 of the shapes below. Show your teacher to prove you have done them. 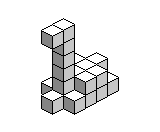 Bench Bench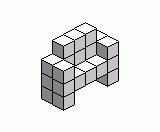 Steamer Aeroplane |
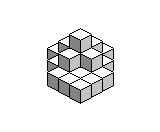 Tunnel
Tunnel