LEARNERS' GRID CONTRACT MATHS A201

Compiled by AnjulberG for non-profit classroom use. Includes material from various sources

HINTS are available for most questions below

INSTRUCTIONS: You must do at least two (2) problems from each module.
Each question is worth either 1, 2 or 3 credits - .
You must score at least 10 credits to complete your contract. You can do more problems to get more credits, of course.

MODULE ONE

(1) Make 24 CREDITS
Use division, multiplication, addition and subtraction to make each set of numbers into equations which will equal 24. You may use brackets, also.

a) 5, 5, 5, 5
b) 2, 4, 6, 7
c) 2, 2, 3, 8
d) 3, 3, 3, 8

(2) Letters, Numbers... Confusion! CREDITS
Look at the equations below. Each letter represents a different digit. Can you figure out which digit each letter represents? [Help]

ABB + CDD = EEE
A - D = C
E - C = A

(3) Farmer Strob

CREDITS

a) Farmer Strob planted a small crop of cabbages. Strob planted 60 rows of cabbages. Each row contained 120 cabbages.
Strob sold his full crop of cabbages for a total of $2808.00.
The costs of fertiliser and seed totalled 8% of what he sold the cabbages for.
How much money did Framer Strob make from EACH cabbage (round off to the nearest cent!)? [Help]
b) Farmer Strob had a few cows, also. He was able to sell 735 litres of milk every seven days.
Strob was paid 32 cents per litre.
During August, the price of milk went up by 15%, which made Strob very happy.
During August, how much MORE did Strob make on milk than he would have in July? [Help]

(4) Mystery Numbers

CREDITS
Name the number for each set of clues.

a) It is a 3-digit whole number. One of the digits is the square root of 16. It's an even number. It's greater than 550. The sum of the digits is 19. Each digit is different. [Help]
b) It is a 3-digit whole number. It's divisible by 4. It is divisible by 5. It's divisible by 11. It's divisible by 3. [Help]
c) It is a 3-digit whole number. It's divisible by 3. It's divisible by 2. It's divisible by 6. The sum of its digits is 12. It is greater than 600. [Help]
d) It is a 4-digit whole number. It's divisible by 4. It's less than 1500. Each of its digits is different. The sum of its digits is 11. Its tens digit is less than its ones digit. The thousands digit is half the tens digit. [Help]

[NOTE: Two correct answers = 1 credit!]

MODULE TWO

(5) How High? 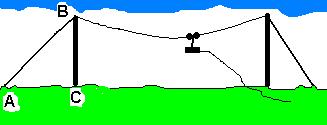CREDITS The above picture shows a flying fox. Look at the angle BÂC. The size of this angle is 50°. The distance from pole to pole is 55 metres. The distance formed by AC is 17 metres. Use a scale drawing to help you calculate the approximate height of the pole BC by using a scale drawing. Show your working.	(6) Some More Soma CREDITS for each puzzle Tower 201 Battleship Steps Wall
(7) Squares and Octagons CREDITS a) Using only a compass, ruler and pencil, construct a perfect square based on a circle and a perpendicular bisection. b) Using only a compass, ruler and pencil, construct a perfect regular octagon based on the technique used above. (An example of a poorly-constructed square and the beginnings of an octagon)	(8) Terrible Tangrams!! CREDITS for every TWO (2) puzzles

MODULE THREE

(9) Problem Solving Galleria. Below are 12 problems.

(Problem 1)
Three puppies and two cats weigh 32 kg. Four puppies and three cats weigh 44kg. All puppies weigh the same and all cats weigh the same. What is the weight of two puppies and one cat? [Help]
(Problem 2)
Find the value of x in each of the problems below:
(a) x – –2 = 13 (b) x + 3 = –3 (c) –7 + x = –15 [Help]
(Problem 3)
It takes one man one day to dig a 2m x 2m x 2m hole. How long does it take 3 men working at the same rate to dig a 4m x 4m x 4m hole? [Help]
(Problem 4)
A farmer grows 252 kilograms of apples. He sells them to a grocer who divides them into 5 kilogram and 2 kilogram bags. If the grocer uses the same number of 5 kg bags as 2kg bags, then how many bags did he use in all? [Help]
(Problem 5)
A cuboid measures 11.3cm x 4.7cm x 12.2cm. What is the weight of this cuboid if the material it is made of weighs 6 grams/cubic centimetre?
(Problem 6)
The area of a rectangular floor is 124.23m². Its length is 12.3m.
(a)  What is the width?  [Help]
(b)  How wide is the door if it is 3% of the perimeter of this room?  [Help]
(Problem 7)
Charlie earns a weekly wage of $652.75. His brother, Derek, earns 21% more per week than Charlie. How much more than Charlie does Derek earn in a year? [Help]
(Problem 8)
Bottles of soft drink were on sale for $8.76 per case of a dozen bottles. If the regular price of each bottle was 97 cents, how much money is saved by buying a case?
(Problem 9)
Plastic sheeting of 0.015cm thickness is rolled onto a circular tube with a 5cm diameter. With the plastic sheeting rolled onto the tube, the diameter of the entire roll is 8.2cm. How many full turns of sheeting are there? [Help]
(Problem 10)
Soglop wants to buy a new car. The price is listed at $33, 500.95 plus GST, but Soglop manages to get a 15% discount on the retail price since the owner of the car dealership was his mother.
How much does Soglop actually pay for the car? [Help]
(Problem 11)
Mrs Jopmeer is pushing a wheelbarrow from her garden to the rubbish bin. The tyre of the wheelbarrow has a radius of 25cm. If the distance from the garden to the rubbish bin is 35 metres, how many times will the wheel rotate completely during this journey? [Help]
(Problem 12)
A 800 seat multiplex is divided into 3 theatres. There are 270 seats in Theatre 1, and there are 150 more seats in Theatre 2 than in Theatre 3. How many seats are in Theatre 2? [Help]

(10) Intergalactic Mining Problems

CREDITS

   The Goolash Intergalactic Mining Corporation has been mining for raw stritomium, a highly volatile but very valuable trans-uranic element, on the planet Yzivesh.
   After one month, the mining station has mined and packaged enough stritomium to fill three transport ships which will take the dangerous but valuable element back to their home planet to be used in constructing hyper-space drives.
   Three transport ships have arrived at Yzivesh under three different commanders: Commander Trox, Commander Wyndax, and Commander Plis. Each transport ship has three special cargo bays, and each bay must contain the same number of Standard Mass Units (SMU) of stritomium and be monitored constantly or their ships will risk serious damage - perhaps even total destruction.

   Commander Trox's ship can carry 910 SMU's in each bay. Commander Wyndax's ship can carry 795 SMU's in each bay. Commander Plis's ship can carry 850 SMU's in each bay.
   The mining crews have arranged 12 Stritomium Containment Units (SCU) on the Loading Field for each commander so that the ship crews can load up their vessels quickly.
   The first problem is, is that there are too many SCUs for each ship. In each case, they will have to leave behind 3 SCUs.
    The second problem is how to load their ships. You must figure out which SCUs each commander must take and work out which SCUs get loaded into which cargo bay.

Below is the table of data each commander was given by the mining station.

Commander Trox	Commander Plis	Commander Wyndax
STRITOMIUM CONTAINMENT UNIT NUMBER/ (SMU)	STRITOMIUM CONTAINMENT UNIT NUMBER/ (SMU)	STRITOMIUM CONTAINMENT UNIT NUMBER/ (SMU)
32t (261 SMU) 41x (610 SMU) 88z (615 SMU) 41a (135 SMU) 50q (90 SMU) 66r (160 SMU) 84y (225 SMU) 91y (110 SMU) 77b (299 SMU) 44c (350 SMU) 62d (120 SMU) 88y (190 SMU)	98v (360 SMU) 42p (25 SMU) 12h (80 SMU) 79m (75 SMU) 53b (345 SMU) 61f (750 SMU) 06j (410 SMU) 22s (110 SMU) 98c (5 SMU) 71w (430 SMU) 13q (95 SMU) 83f (30 SMU)	37j (35 SMU) 47k (265 SMU) 91v (235 SMU) 99n (85 SMU) 56c (215 SMU) 63z (295 SMU) 18t (195 SMU) 20h (705 SMU) 11g (275 SMU) 08z (325 SMU) 60y (25 SMU) 28s (55 SMU)

Get the answers here.