LEARNERS' GRID CONTRACT MATHS B101

Compiled by AnjulberG for non-profit classroom use. Includes material from various sources

HINTS are available for most questions below

INSTRUCTIONS: You must do at least two (2) problems from each module.
Each question is worth either 1, 2 or 3 credits - .
You must score at least 10 credits to complete your contract. You can do more problems to get more credits, of course.

MODULE ONE

(1) Make 24 CREDIT Use division, multiplication, addition and subtraction to make each set of numbers into equations which will equal 24. You may use brackets, also. a) 9, 4, 6, 8 b) 1, 5, 6, 6 c) 3, 3, 6, 9 d) 2, 3, 9, 9	(2) Letters, Numbers... Confusion! CREDIT Look at the equations below. Each letter represents a different digit. Can you figure out which digit each letter represents? [Help] ABC + DEF = GEG A - E = E F - C = E
(3) PATTERNS CREDIT for 3 completions a) 1, 3, 6, 10, ______, ______, ______ b) 16, 10, 5, 1, ______, ______, ______ c) J, F, M, A, ______, ______, ______ d) (d,1), (c,-1), (b,-3), (a,-5), ___, ___, ___	(4) COUNTRY DRIVE CREDIT Lucy was driving through the countryside. She saw a farm scene where she counted 24 heads and 92 legs. There were dogs, sheep and people. If there were ten times as many sheep as there were dogs, how many of each of the three were there?
(5) CARD COLLECTION CREDIT Jack and Janice collect wrestling cards. In total, they have 171. If Janice has twice as many as Jack, how many more cards does she have?	(6) WHO'S WHO IN QUEUE? CREDIT A groups of friends are standing in line to buy sports tickets. May and Tim were standing next to each other. Maddy was at one end of the group. Greg was in front of Sue and Maddy, but behind May. Tim is not first. Name the order in which these friends were lined.

MODULE TWO

(7) SHIFTING SHAPES 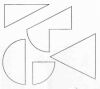CREDIT Cut out the shapes on the left to form a heart shape. Your teacher has copies of larger shapes for you to use. If you are online, go here to get a bigger copy of these shapes.	(8) Some More Soma 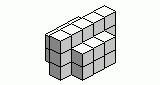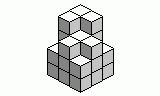CREDIT for each puzzle SlabsMartian House
(9) NETS CREDIT Draw three (3) different nets which could be folded to form a cube.	(10) Pentomino Puzzler!! CREDIT Draw a 6 x 5 grid. Next, show how you could perfectly arrange 6 different pentominoes inside this grid.

MODULE THREE

(11) Problem Solving Galleria. Below are 10 problems. Show your working to have your answers considered completed.

(Problem 11.1) Cameron is building a fence. He puts in five posts in a straight line along the boundary, making sure the posts are 5m apart. How far is it from the first post to the last post? [Help]
(Problem 11.2) Find the value of x in each of the problems below: (a) x + 2 = – 33 (b) x – –3 = –3 (c) –21 ÷ x = –3 [Help]
(Problem 11.3) A box has a volume of 1 cubic metre. It is then packed with wooden die. Each dice measures 1cm x 1cm x 1cm. If all these small cubes were taken out and placed end-to-end in a long line, how far would it stretch? Give your answers in kilometres. [Help]
(Problem 11.4) A farmer grows 420 kilograms of apples. He sells them to a grocer who divides them into 5 kilogram and 2 kilogram bags. If the grocer uses the same number of 5 kg bags as 2kg bags, then how many bags did he use in all? [Help]
(Problem 11.5) A cuboid measures 11.5cm x 4.7cm x 12.5cm. What is the weight of this cuboid if the material it is made of weighs 6 grams/cubic centimetre? Give your answer in kilograms.
(Problem 11.6) A set of 5 tennis balls normally sells for $5.20. If they are discounted by 25%, what is the sale price of each tennis ball?
(Problem 11.7) Jiro earns a weekly wage of $660.75. His brother, Jun, earns 25% more per week than Jiro. How much more than Jiro does Jun earn in a year? [Help]
(Problem 11.8) Bottles of soft drink were on sale for $13.20 per case of two dozen bottles. If the regular price of each bottle was 68 cents, how much money is saved by buying a case?
(Problem 11.9) Plastic sheeting of 0.015cm thickness is rolled onto a circular tube with a 5cm diameter. With the plastic sheeting rolled onto the tube, the diameter of the entire roll is 7.2cm. How many full turns of sheeting are there? [Help]
(Problem 11.10) Mrs Haas is pushing a wheelbarrow from her garden to the rubbish bin. The tyre of the wheelbarrow has a radius of 18cm. If the distance from the garden to the rubbish bin is 35 metres, how many times will the wheel rotate completely during this journey? [Help]

Get the answers here.